QED

Feynman Graphs

  1. Interaction of an electron with an external electromagnetic field
  2. Electron Muon scattering
  3. The Feynman Rules
    In the following the Feynman rules which have been derived in the last section are summarized. To calculate the T-matrix element a Feynman diagram, representing the amplitude to a given order in the perturbation series, can drawn. The Feynman diagram to first order for electron-muon scattering is given by
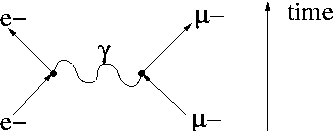
    electronmuonscattering
    All elements of the diagram, that is, external lines, internal lines and vertex factors have to be multiplied. For fermions and photons these factors are tabulated here:
    feynmanrules
    An integration over internal momenta is implied.


    ADDING AMPLITUDES
    If several diagrams contribute the corresponding amplitudes have to be added.
    For identical fermions these amplitudes have to be subtracted.


    SUMMING OVER SPINS

    Often, the incoming particle beam is unpolarized, and the polarization of the outgoing particles is not measured. In this case, one has to average over the spins (helicities) of the intial state and to sum over the spins (helicities) of the final state.

    In this case, the spin averaged amplitude squared has to be formed when calculating rates or cross sections:
    spinaveragedamplitudesquared
    This can be written as
    spinaveragedamplitudesquared_1
    where
    electronmuontensor


    That is, one needs to know trace theorems of the various kinds to evaluate spin averaged amplitudes squared in an analytical way.

    To evaluate Feynman diagrams on a computer it is much more convenient to calculate each of the 16 terms indivudually and sum them up.